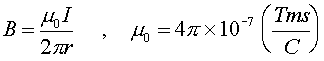Materials underpin 70% of the GNP of the industrialised nations, in one way or another, and are therefore vital to the economy. Nanotechnology provides a route to the creation of almost limitless kinds of novel materials in a variety of ways. Nanomaterials can be described as 'novel materials whose size of elemental structure has been engineered at the nanometre scale'. Materials in the nanometre size range exhibit fundamentally new behaviour, as their size falls below the critical length scale associated with any given property. Intervention in the properties of materials at the nanoscale permits the creation of materials and devices with hitherto undreamed of performance characteristics and functionality.

Classification and Composition of Nanomaterials

Nanomaterials include clusters of atoms (quantum dots, nanodots, inorganic macromolecules), grains that are less than 100 nanometres in size (nanocrystalline, nanophase, nanostructured materials), fibres that are less than 100 nanometres in diameter (nanorods, nanoplatelets, nanotubes, nanofibrils, quantum wires), films that are less than 100 nanometres in thickness, nanoholes, and composites that are a combination of these. The composition can be any combination of naturally occurring elements, with the more important compositions being silicates, carbides, nitrides, oxides, borides, selenides, tellurides, sulfides, halides, alloys, intermetallics, metals, organic polymers, and composites.

Magnetic Resonance in an Atomic Vapor Excited by a Mechanical Resonator

For the first time, mechanical motion has been used to make atoms in a gas "spin," scientists at the National Institute of Standards and Technology (NIST) report. The technique eventually might be used in high-performance magnetic sensors, enable power-efficient chip-scale atomic devices such as clocks, or serve as components for manipulating bits of information in quantum computers.

As described in the Dec. 1 issue of Physical Review Letters, the NIST te 13213j99n am used a vibrating microscale cantilever, a tiny plank anchored at one end like a diving board, to drive magnetic oscillations in rubidium atoms. The scientists attached a tiny magnetic particle about 10 by 50 by 100 micrometers in size'to the cantilever tip and applied electrical signals at the cantilever's "resonant" frequency to make the tip of the cantilever, and hence the magnetic particle, vibrate up and down. The vibrating particle in turn generated an oscillating magnetic field that impinged on atoms confined inside a 1-square-millimeter container nearby.

The electrons in the atoms, acting like tiny bar magnets with north and south poles, responded by rotating about a static magnetic field applied to the experimental set-up, causing the atoms to rotate like spinning tops that are wobbling slightly. The scientists detected the rotation by monitoring patterns in the amount of infrared laser light absorbed by the spinning atoms as their orientation fluctuated with the magnetic gyrations. Atoms absorb polarized light depending on their orientation with respect to the light beam.

Micro-cantilevers are a focus of intensive research in part because they can be operated with low power, such as from a battery, and yet are sensitive enough to detect very slight changes in magnetic fields with high spatial resolution. The NIST team noted that coupling between cantilever motion and atomic spins is easy to detect, and that the atoms maintain consistent rotation patterns for a sufficiently long time, on the order of milliseconds, to be useful in precision applications.

For instance, by comparing the oscillation frequency of the cantilever to the natural rotation behavior of the atoms (determined by measuring the extent of the wobble), the local magnetic field can be determined with high precision. Or, arrays of magnetic cantilevers might be constructed, with each cantilever coupled vibrationally to the others and coupled magnetically to a unique collection of atoms. Such a device could be used to store or manipulate binary data in a quantum computer. In theory, the coupling process also could work backwards, so that atomic spins could be detected by monitoring the vibrational motion of the cantilevers.

https://www.nist.gov

Magnetic field of a long wire

Magnetic fields arise from charges, similarly to electric fields, but are different in that the charges must be moving. A long straight wire carrying a current is the simplest example of a moving charge that generates a magnetic field. We mentioned that the force a charge felt when moving through a magnetic field depended on the right-hand rule. The direction of the magnetic field due to moving charges will also depend on the right hand rule. For the case of a long straight wire carrying a current I, the magnetic field lines wrap around the wire. By pointing one's right thumb along the direction of the current, the direction of the magnetic field can by found by curving one's fingers around the wire.

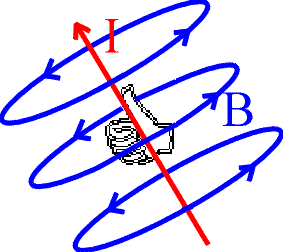
The strength of the magnetic field depends on the current I in the wire and r, the distance from the wire.

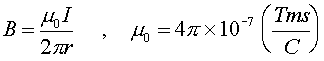
The constant m is the magnetic permiability. The reason is does not appear as an arbitray number is that the units of charge and current (coulombs and amps) were chosen to give a simple form for this constant. One can also notice the the product of m and e are related to the velocity of light. (More on that later, fundamental constants

Nanofoam Exhibits Surprising Magnetic Properties

By Ernie Tretkoff

A new form of carbon exhibits surprising magnetic properties that could make it useful in future spintronics or biomedical applications, researchers reported at the APS March Meeting. The material, called carbon nanofoam for its low density and web-like structure, is the only form of pure carbon known to be ferromagnetic.

Carbon nanofoam is structurally distinct from the other four known forms of carbon-graphite, diamond, fullerenes ( buckyballs), and nanotubes. With a density of about 2 mg/cm³, comparable to that of aerogel, carbon nanofoam is one of the lightest known solid substances.

But what's most remarkable about the material, the researchers said, is that unlike other forms of carbon, the nanofoam is ferromagnetic, like a refrigerator magnet. However, at room temperature, the nanofoam's magnetization disappears a few hours after the material is produced.

A collaboration of researchers from Greece and Australia produced the carbon nanofoam by shooting a high-powered, ultra-fast laser at disordered solid carbon in an argon-filled chamber.

By imaging the material using a high-resolution electron microscope, John Giapintzakis of the University of Crete and colleagues found that the nanofoam has a sponge-like structure, made up of carbon clusters a few nanometers in diameter randomly linked together into a web-like foam.

Because pure carbon is not normally ferromagnetic, the group tested their sample for impurities that might be causing the magnetic behavior. Although they did find traces of iron and nickel, the small amounts of these magnetic elements could not account for all of the ferromagnetism in the nanofoam. The researchers concluded that the magnetic properties come from the complex structure of the nanofoam itself.

David Tománek of Michigan State University, who collaborated with the group on theoretical interpretation, believes that the carbon clusters in the foam are made up of nanotubes joined together into tetrapods. In these four-legged structures, some carbon atoms have a free electron, one that does not form a chemical bond. These unpaired electrons carry a magnetic moment that may lead to the magnetism.

Chemists have long known about such carbon radicals, said Tománek, but until now they have only been found in carbon connected to another element. In this case, the structure is entirely carbon.

The researchers have also done some preliminary studies that suggest that the novel magnetic behavior found in carbon nanofoam could be present in other nano-structured solids of elements that are not normally magnetic, including a compound of boron and nitrogen.

If this behavior turns out to be a general phenomenon, researchers will have to think more about what makes a material magnetic, said Tománek. "We need to revisit our magnetic prejudice."

Giapintzakis suggested that carbon nanofoam could be used in spintronic devices, which are based on a material's magnetic properties. The unique material may also find uses in biomedicine. For instance, the tiny ferromagnetic clusters could be injected into blood vessels to enhance magnetic resonance imaging. The nanofoam could also be implanted in tumors, where it could turn radio waves into a source of heat that would destroy the tumor but leave surrounding tissue unharmed.

Circular motion in a magnetic field

Charged particles in a magnetic field feel a force perpendicular to their velocity. Since their movement is always perpendicular to the force, magnetic forces due no work and the particle's velocity stays constant. Since the force is F = qvB in a constant magnetic field, a charged particle feels a force of constant magnitude always directed perpendicular to its motion. The result is a circular orbit.

The diagram below represents constant magnetic field for two cases. On the left the magnetic field is pointed into the page while on the right the field lines are exiting the page. The crosses indicate the field is directed into the page. One can think of this as the tail of a feather as it travels away from view, whereas the dots represent the point of the approaching arrow. The fact that the field is uniform is indicated by the equal spacing of the arrows. Using the right-hand rule one can see that a positive particle will have the counter-clockwise and clockwise orbits shown below.

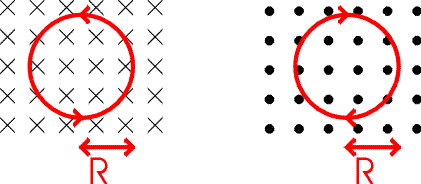
The radius of the orbit depends on the charge and velocity of the particle as well as the strength of the magnetic field. The acceleration of a particle in a circular orbit is:

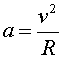
Using F = ma, one obtains:

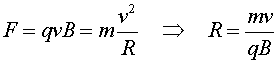
Thus the radius of the orbit depends on the particle's momentum, mv, and the product of the charge and strength of the magnetic field. Thus by measuring the curvature of a particle's track in a known magnetic field, one can infer the particle's momentum if one knows the particle's charge. A device which works in such a fashion is called a magnetic spectrometer. Most high-energy physics experiments use exactly such devices, even though the particle's have so much momentum that they never circle but only curve a few degrees from their straight line trajectories.

Magnetic dipoles

A magnetic dipole is simply a current loop. A current loop both creates and responds to magnetic fields. First let's review the torque felt by a dipole in a magnetic field. Consider the current loop in a magnetic filed below. The two forces are equal and opposite and do not act to move the dipole, only to rotate it.

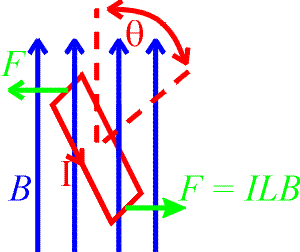
The moment arm for the torque is the height of the dipole, h sinq. the angle q is between the direction of the magnetic field and a vector normal to the plane of the dipole. Therefore the torque disappears when the dipoles orientation is along the axis of the magnetic field (the dipole lies in a horizontal plane in the picture above.) The torque always tries to rotate the angle q to zero. This is where the potential energy is a minimum. The torque and potential energy are:

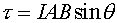
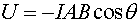
where A is the area of the dipole's loop. The product IA is refered to as the dipole moment. Magnetic, non-magnetic, ferromagnetic....

Many atoms have electrons which are able to rotate any way they please. These rotating electrons are the dipoles which both create and respond to magnetic fields. Consider the magnetic field of the red dipole shown below.

A second dipole, shown in green will find it energetically favorable to line up in the same direction as the red dipole if it is placed directly above it, but will find it favorable to line up opposite to the dipole if it is place to the side. The study of properites is therefore a rather complex subject.

There are a variety of classifications of magnetic materials.

Here we list three such classifications.

Magnetic Quantum Wells and Magnetoelectronics

The state of the art in surface preparation and analysis has made it feasible to produce new types of materials that are structured on the nanometer scale. This scale is comparable to the wavelength of electrons in a solid and thus provides ample opportunity to design the electronic and magnetic properties. We can think of designing solids in a fashion similar to the design of molecules and drugs in biochemistry. Some of the best examples of engineered solids so far are magnetic multilayers that are being developed for reading heads for magnetically-stored data. They consist of sandwiches of cobalt, copper, and permalloy (nickel-iron) that change their electrical resistance when exposed to the magnetic field of a stored bit. This effect is termed giant magnetoresistance (GMR). The structures are known as spin valves, since they preferentially transmit electrons of one spin orientation. A related phenomenon is oscillatory magnetic coupling, an oscillation in the magnetic orientation of two layers with film thickness.

To find out which electrons are producing these effects we have investigated their energy levels by inverse photoemission. Thereby, low energy electrons impinge onto the surface and drop into unoccupied energy levels emitting ultraviolet photons. This study discovered quantized electronic states in magnetic multilayers that are connected to their special properties.

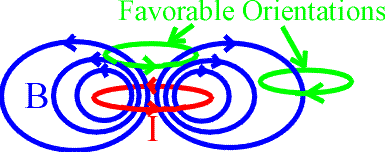
The figure shows periodic changes in the density of electron states when the thickness of a copper film is changed, just a couple of atomic layers at a time (top panel). A way of understanding the experiment is shown on the right: Electrons in the Cu film are acting like waves and give maximum intensity when an integer number of oscillations fits into the film. These structures can be viewed as the smallest man-made interferometers, right at the atomic limit. They allow us to map out the wave function of electrons.

It turns out that the density-of-states maxima are correlated with oscillations of the magnetic coupling in multilayers (bottom panel, from Qiu et al., Phys. Rev. B 46, 8659 (1992)). The magnetoresistance oscillates with the same period. This gives us the clues for understanding the magnetic phenomena as the effects of quantized electron levels in nanostructures. Current efforts are directed towards manipulating the interfaces in magnetic multilayers to enhance the spin-dependent reflectivity and optimize the magnetic effects.

Review of magnetic nanostructures:
Himpsel et al., Advances in Physics 47, 511 (1998).

Basic principles of magnetic recording:
Grochowski and Thompson, IEEE Trans. Magn. 30, 3797 (1994)

Magnetic Quantum Well States::
Ortega et al., Phys. Rev. Lett. 69, 844 (1992); Ortega et al., Phys. Rev. B 47, 1540 (1993); Himpsel, Science 283, 1655 (1999).

Writing and Reading of a Hard Disk
Schematic of a GMR Reading Head
Explanation of GMR by Analogy to a Pair of Polarizers
Spectra of Quantum Well States
Oscillations in the Density of States
Spin-polarization of quantum well states

Electronic States in Magnetoelectronics

Beyond the GMR effect in reading heads there are several other magnetic phenomena that can be incorporated into electronic devices. For example, spin-polarized tunneling lies at the core of magnetic random access memory (MRAM). A new field of magnetoelectronics is developing, where spin currents are used instead of charge currents. For that, it is important to know how to produce spin-polarized electrons, how to filter them, and how to detect their spin. Electrical and magnetic measurements provide practical information, but they integrate over all momenta, that is, they do not distinguish between electrons moving in different directions and at different speeds.

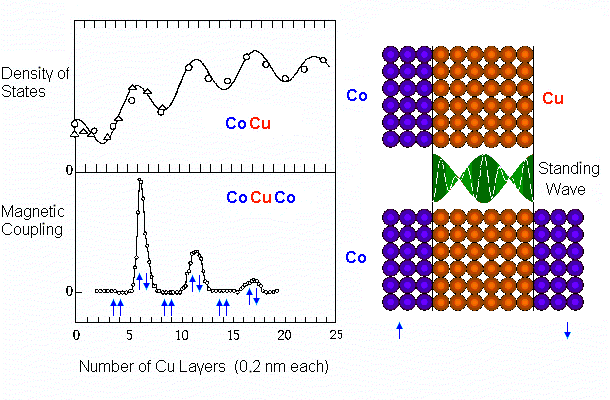
The cleanest technique to resolve the momenta of these electrons is angle-resolved photoelectron spectroscopy, which is able to measure the complete set of quantum numbers of electrons in solids. The figure below shows a theoretical plot of the quantum numbers energy (E) and momentum (k) for the two spin directions (red and green). The photoemission experiment on the right zooms in on the electrons that are relevant to magnetoelectronics. These are those close to the Fermi level (E=0). The intensity of these electrons is plotted versus their momentum in the two panels at the bottom right. From the k-separation of the two spin peaks one can infer the magnetic moment, from their relative intensity the spin-polarization, and from their width the mean free path of the electrons.

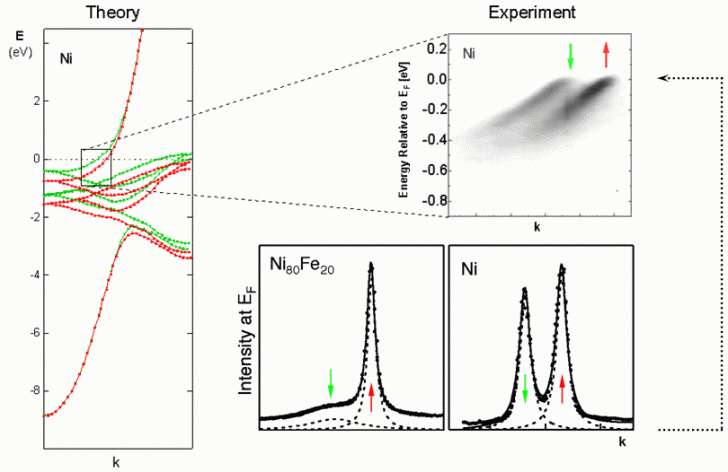
Spin currents can be manipulated by spin doping, where magnetic atoms are introduced as dopants for selecting one type of spin carrier. For example, adding iron to nickel suppresses the green spins, but not the red spins in the figure above. The Fe/Ni ratio of 20/80 corresponds to permalloy, the dominant material in magnetoelectronics.

Metal-based magneto-electronics

This research aims at using the properties of ferromagnetic materials to realize `More than Moore' functionality on a chip. Different concepts were investigated where hetero-integration of ferromagnetic thin films, multi-layers and nanostructures on top of conventional semiconductors results in new devices for a wide range of applications such as next-generation magnetic memories, RF components, sensors and magnetophotonic components C11041 .

Voltage-controlled magnetic memories

Writing magnetic information using on-chip generated magnetic fields typically requires very high currents. IMEC has demonstrated proof of principle of voltage-tunable magnetic properties in a hybrid piezomagnetic device based on the shear actuation by ferromagnetic electrodes on a piezoelectric substrate. This device allows influencing the magnetization reversal process using a voltage through the inverse magnetostrictive effect. Figure1 shows a voltage-induced change in magnetoresistance of Co₅₀Fe₅₀ interdigitated electrodes on piezoelectric lead zirconate titanate (PZT) at zero magnetic field. The magnetic anisotropy and switching field can be changed with a factor 2 using solely electric fields of around 5kV/cm. Miniaturization and geometric optimization of this concept opens a way to low-voltage and low-power magnetic reversal in magnetic devices used in sensor and memory applications

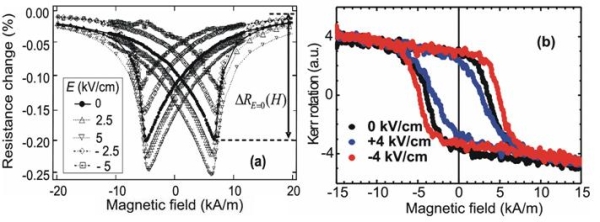
Figure 1: The magneto-resistance of 10nm thick Co₅₀Fe₅₀ interdigitated electrodes can be tuned by a bias voltage applied across two sets of ferromagnetic electrodes (separation 200µm) deposited on a piezoelectric lead zirconate titanate (PZT) substrate. (b) The voltage-induced change in magnetic anisotropy, revealing the change in the switching field, is confirmed by the evolution of the magnetic hysteresis loops (obtained by magneto-optical Kerr effect measurements).

Current-controlled spin torque oscillators

The recent discovery of the spin torque oscillator, a nano-patterned ferromagnetic device in which high-quality tunable microwave oscillations can be generated by a small DC current through the device, opens perspectives to solve some of the paradigms in microwave engineering. None of the RF oscillators existing today combines a high-quality resonance with a high integration level, and wideband tunability. This year, IMEC has started fabrication of spin torque oscillators made from magneto-resistive spin valves with typical sizes around 100nm. IMEC aims at studying the oscillating modes in the range of 5-10GHz and the influence of parameters (e.g. temperature, geometry) on the microwave frequency, signal power and phase noise. Monolithic integration with a high-gain RF-CMOS amplifier circuit will boost the signal power to levels suitable for wireless applications. RP112

Cell counting device based on magneto-resistive sensors

Over the last years, IMEC has successfully applied magneto-resistive spin valve sensors for magnetic particle labeled biomolecule detection P11053, RP030 . Until now, these efforts have focused on detecting specific markers and DNA using assays, see also (see section Functionalized particles).

For the purpose of rare cell detection using the same magneto-resistive technology, we have studied the movement of magnetic particles actuated by an on-chip multi-phase magnetic field. Magnetic particles with different magnetophoretic mobility are successfully separated on a chip and their magnetoresistive signals are detected with single-particle resolution. In addition, the specific binding of magnetic particles and target cells, to isolate rare cells out of a large population is currently being investigated.

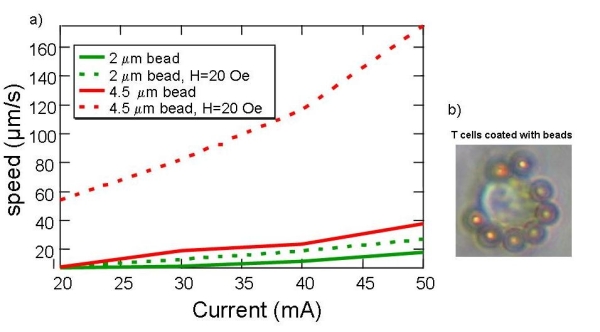
Figure 2: (a) Velocity of two types of magnetic particles at different actuating current. Green lines: speed curve of industrial-type1 2μm particles (D=2μm); red lines: velocity curve of industrial-type2 particles (D=4.5μm). Solid lines: velocity curve with only on-chip magnetic field; dashed lines: velocity curve with 160A/m perpendicular-to-plane magnetic field. (b) A molt-4 T-lymphocyte bound industrial-type CD45 magnetic particles.

Nano-electromechanical sensors (NEMS)

Nano-electromechanical systems offer immense potential for sensor technology requiring ultimate sensitivity. Nanoresonators used as force and mass sensors have shown unprecedented sensing potential: in force sensing, a nanomechanical resonator used as a magnetic-force microscope was able to detect the magnetic moment of a single electron spin; in mass sensing, researchers were able to detect a mass of 7 zeptogram (equivalent to 30 Xe atoms) using a nanomechanical resonator.

At IMEC, nanomechanical resonators made from diamond, AlGaAs and silicon (Figure3) were investigated. The shape dependence of the mass sensitivity of nanomechanical resonators was studied: silicon resonators were fabricated with a novel `double-triangular' cross-section leading to enhanced mass-sensing capabilities C11046.

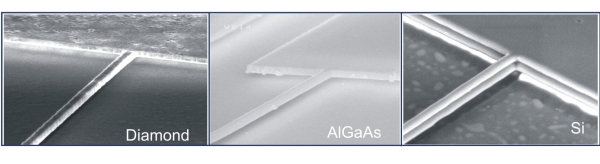
Figure 3: Nanomechanical resonators fabricated in different materials: diamond, AlGaAs and silicon. The width of the devices is typically 200 nm. The silicon device on the right has a novel `double-triangular' cross-section leading to enhanced mass-sensing capabilities.

Magnetophotonic components

In close collaboration with UGent/INTEC a magneto-optical waveguide device was demonstrated, based on ferromagnetic contacts on top of III-V-based optical amplifier with 104dB/cm non-reciprocal propagation. This device can be used as an isolator that can be monolithically integrated with a conventional DFB laser. RP063, C11054 (see section Applications in photonic systems

Document Info

Accesari: 2218
Apreciat:

Comenteaza documentul:

Nu esti inregistrat
Trebuie sa fii utilizator inregistrat pentru a putea comenta

Creaza cont nou

A fost util?

Daca documentul a fost util si crezi ca merita
sa adaugi un link catre el la tine in site

Copiaza codul:
in pagina web a site-ului tau.

eCoduri.com - coduri postale, contabile, CAEN sau bancare

Politica de confidentialitate | Termenii si conditii de utilizare